ProblemIn the square below, each vertex is joined to the midpoint of the opposite sides and a star shape is formed.  What fraction of the square is shaded? SolutionConsider the following unit. 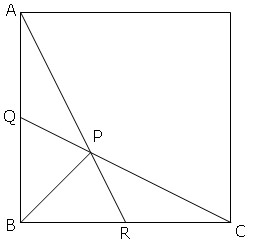 As $R$ is the midpoint of $BC$, length $BR$ = length $RC$, and as $\Delta BPR$ and $\Delta RPC$ have the same altitude they must have the same area. In the same way, $\Delta APQ$ and $\Delta QPB$ have the same area. Therefore, $A = A \Delta APQ = A \Delta QPB = A \Delta BPR = A \Delta RPC$. As $A \Delta BAR = 1/4$ of the square, it follows that $3A = 1/4 \Rightarrow A = 1/12$. Thus the area of quadrilateral $BQPR = 2A = 1/6$, and we can see that in the original diagram $4 \times 2A = 8A = 8/12 = 2/3$ is unshaded. Hence the shaded "star" represents $1/3$ of the area of the square.
|