Oplossing week 17

Oplossing 1

---

Met de stelling van Ptolemaeus geeft dat:

$ \eqalign{ & \sqrt {60} \cdot \sqrt {64 - x^2 } = 2 \cdot 8 + 2x \cr & \sqrt {3840 - 60x^2 } = 2x + 16 \cr & 3840 - 60x^2 = 4x^2 + 64x + 256 \cr & 64x^2 + 64x - 3584 = 0 \cr & x^2 + x - 56 = 0 \cr & (x + 8)(x - 7) = 0 \cr & x = - 8\,\,(v.n.)\,\, \vee x = 7 \cr & x = 7 \cr} $

Maar dat kan vast ook anders...

---

Oplossing 2

---

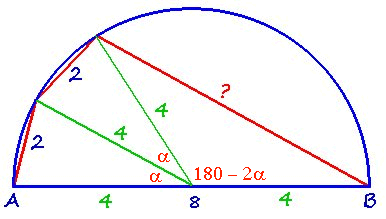
Gebruik de cosinusregel:

$
\eqalign{
  & 2^2  = 4^2  + 4^2  - 2 \cdot 4 \cdot 4 \cdot \cos \alpha   \cr
  & \cos \alpha  = \frac{7}
{8}  \cr
  & \cos 2\alpha  = 2\cos ^2 \alpha  - 1  \cr
  & \cos 2\alpha  = \frac{{17}}
{{32}} \cr}
$

Nogmaals de cosinusregel:

$
\eqalign{
  & x^2  = 16 + 16 - 2 \cdot 4 \cdot 4 \cdot \cos (180 - 2\alpha )  \cr
  & x^2  = 32 - 32 \cdot  - \frac{{17}}
{{32}}  \cr
  & x^2  = 49  \cr
  & x = 7 \cr}
$

Ook mooi...

---

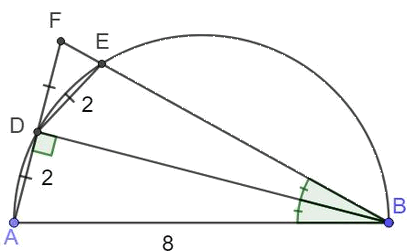
Oplossing 3

---

Met dank aan Erik:

©2004-2024 W.v.Ravenstein